Chapter 6: Device driver, Local variables, and LCD output
Jonathan Valvano and Ramesh Yerraballi
In this chapter we will learn how to allocate local variables on the stack. Variables are an important concept in programming. Scope defines where in the software a variable can be accessed. Allocation define how the variable is implemented. If the variable needs to be permanent, it will be placed in RAM. If the variable is temporary, we can allocate it in a register or on the stack.
The second objective of this chapter is to interface an LCD to the microcontroller and write a set of functions to output numbers and strings to the display. We will use fixed-point numbers to specify non-integer values using integer math. We will introduce recursion as a software design technique.
Table of Contents:
- 6.1.
Invocation, Declaration, and Definition of Functions
- 6.2.
Local Variables
- 6.3.
Stack frames
- 6.4. Linking C to assembly
- 6.5. Serial Peripheral Interface (SPI)
- 6.6. ST7735R Interfacing
- 6.7.
Fixed-point Numbers
- 6.8.
Numerical Output
- 6.9.
ECE319K Lab 6 Videos
Return to book table of contents
Video 6.0. Introduction to Chapter 6, and ECE319K Lab 6.
6.1. Invocation, Declaration, and Definition of Functions
Before discussing local variables, let's review functions. A software module has three parts.
- A higher-level file with software that calls public functions of the module
- A header file with prototypes for the public functions of the module
- A code file with definitions for the public and private functions of the module
Video 6.1.1. Modular approach to software development.
An invocation is where the function is called. The caller establishes the input parameters and executes a BL to the function. A prototype or declaration defines the function name and the number/types of the input/output parameters. The definition the actual code that will be executed. In general, The function invocations exist at a higher level than the definitions. Typically, the function prototypes or declarations are in a header file, and the function definitions are in a code file. The video outlines this modular approach to software development Program 6.1.1 shows the main.c file, which includes the function invocations. Program 6.1.2 shows the Logger.c file, which includes the function definitions. Program 6.1.3 shows the Logger.h file, which includes the function declarations..
// main.c
#include "UART.h"
#include "random.h"
#include "Logger.h"
// Global Variables in RAM
char *progtitle="Histogram of Randoms"; // Global Scope and
// Permanent persistence (RAM)
// The entry point is a function with global scope
int main(){
uint32_t i; // Local scope (in main) and persists
// as long as main does does not return
// allocated on the Stack
Output_Init();
Random_Init(1317); // Initialize the Random Number
Generator
for (i=0; i < 100; i++){
uint32_t val; // Local scope (for loop), and
persists
// while the for loop runs
// allocated on the Stack
val = Random();
Logger_track(val%MAXVAL);
}
Logger_display();
while(1);
}
Program 6.1.1. The main.c file used in the above video.
// Logger.c
// Keeps track of the frequencies of values in a local array
// and displays them like a histogram when requested
#include <stdio.h>
#include "Logger.h"
#define LineWidth 40
static uint8_t Frequency[MAXVAL]; // Local scope (to file Logger.c)
// permanent
persistence (RAM)
extern char *progtitle;
static void pretty_print(uint8_t, uint8_t); //Prototype
static void LogInit(){
uint8_t i; // Local scope (in LogInit) and persists
// as long as LogInit does does not return;
// allocated on the Stack
for (i=0; i < MAXVAL; i++)
Frequency[i]=0;
}
// Keeps track of values on successive calls
// in the Log array
uint8_t Logger_track(uint32_t val){
static uint8_t first=0; // Local (in Logger_track)
// permanent persistence (RAM)
if(first == 0){
LogInit();
first=1;
}
if(val > MAXVAL) return(0); // Error check - fail
Frequency[val]++; // Increment frequency of the value
return(1); //success
}
void Logger_display(){
uint8_t index;
printf("%s\n",progtitle);
for (index=0; index< MAXVAL; index++){
pretty_print(index,Frequency[index]);
}
}
// Local (to file) static function that can only be called from
// within this file
static void pretty_print(uint8_t val, uint8_t times){
uint8_t i;
printf("%d:",val);
for(i=0; i < times; i++){
if (i >= LineWidth) break;
printf("*");
}
printf("%d\n",times);
}
Program 6.1.2. The Logger.c code file used in the above video.
// Logger.h
uint8_t Logger_track(uint32_t val); // log val
void Logger_display(); // display the data
Program 6.1.3. The Logger.h header file used in the above video.
:What does the static means in "static uint8_t Frequency[MAXVAL]"?
:What does the extern mean in "extern char *progtitle"?
: What does the static means in "static void LogInit()"?
: What does the static means in "static uint8_t first=0"?
: Why does pretty_print have a prototype, but LogInit does not?
6.2. Local Variables
Variables are an important component of software design, and there are many factors to consider when creating variables. Some of the obvious considerations are the size and format of the data. In this class we will consider integers, which can be 8-bit, 16-bit or 32 bits. Furthermore, integers can signed or unsigned. Table 6.2.1 shows the C99 type definitions.
| Precision | Unsigned | Signed |
|---|---|---|
| 8 bits | uint8_t | int8_t |
| 16 bits | uint16_t | int16_t |
| 32 bits | uint32_t | int32_t |
Table 6.2.1. C99 type definitions for integers.
Another factor is the scope of a variable. The scope of a variable defines which software modules can access the data. Variables with an access that is restricted less than everywhere are classified as private, and variables shared between multiple modules are public. In general, a system is easier to design (because the modules are smaller and simpler), easier to change (because code can be reused), and easier to verify (because interactions between modules are well-defined) when we limit the scope of our variables. However, since modules are not completely independent we need a mechanism to transfer information from one to another. The ARM Application Binary Interface (ABI) has detailed descriptions of how to develop software interfaces. However, in this chapter, we will discuss the fundamentals of software interfaces.
An addition consideration for variables is allocation
or persistence. We could place variables in registers temporarily,
on the stack in RAM temporarily, in RAM permanently, or in ROM
permanently. We will use the terms allocated permanently and permanent
persistence to mean the same thing, created at compile time and never
destroyed. Because their contents are allowed to change, all variables
must be allocated in registers or RAM and not ROM. Constants can be placed
in ROM. A local variable has reduced scope and temporary
allocation. We can allocate a local variable in a register or on the
stack. One of the important objectives of this chapter is to present
design steps for creating, using, and destroying local variables on the
stack. In C, we create a local variable by defining it within the
function. We will consider parameters passed into or out of a function as
local variables, because they have reduced scope and temporary allocation.
The scope of the variable sum is within the entire
function, whereas the scope of i is within the
for-loop. Local variables are not initialized. Therefore it is your
responsibility to initialize your local variables.
While reading the following examples, notice the scope and allocation of the different variables. There are two
separate variables called num.
| Variable | Classification | Scope | Allocation |
|---|---|---|---|
| sum | local | MyFunction | stack |
| i | local | for-loop | stack |
| TotalCount | static | that file | RAM |
| num | static | MyFunction2 | RAM |
| num | static | MyFunction3 | RAM |
| flag | global | everywhere | RAM |
Table 6.2.2. Scope and allocation
uint32_t MyFunction(void){uint32_t sum;
sum = 0;
for(uint32_t i=0; i < 10; i++){
sum=sum+i;
}
return sum;
}
A static variable has reduced scope and
permanent persistence. The compiler allocates static variables in permanent RAM. The
scope can be reduced to a single function or a single file. Static
variables will be initialized to 0 on software reset, or we can explicitly
initialize it. It is good programming practice to initialize all your
varaibles, even if the compiler does initialize them to 0. Static
variables are initialized just once, at reset. In this example TotalCount
is initialized once to 0, it is shared within the file, so accessible to
both functions. TotalCount contains the total number
of times either function has been called. There are two copies of Num,
one for each function. The static Num variable
maintains the number of times each function has been called. The two
functions will return 1 if that function has been called more than 75
times or if the sum of the two calls is more than 100.
static uint32_t TotalCount=0;
uint32_t MyFunction2(void){
static uint32_t Num=0;
Num++; TotalCount++;
if((Num > 75)||(Count > 100)){
return 1; }
return 0;
}
uint32_t MyFunction3(void){
static uint32_t Num=0;
Num++; TotalCount++;
if((Num > 75)||(Count > 100)){
return 1; }
return 0;
}
A global variable has public scope and
permanent persistence. Public scope means any software in the system has
access to the variable. Global variables are permanently allocated in RAM.
Global variables will be initialized to 0 on software reset, unless we can
explicitly initialize it to something else. We will consider I/O port
registers as global variables, because they have public scope and
permanent persistence. The global variable Flag can
be accessed by both MyFunction4 and MyFunction5,
even if the functions are in different files. The extern
definition does not create a second copy of the variable, rather, it
provides access to the single shared global. Assume Flag and MyFunction4 are in one file.
uint32_t Flag;
void MyFunction4(void){
Flag = 0;
}
Assume MyFunction5 is in a different file than Flag and MyFunction4.
extern uint32_t Flag;
void MyFunction5(void){
Flag = 1;
}
Observation: It is poor programming style to use extern because it creates difficult to manage coupling between two modules.
In general, the qualifier const added to a
variable definition means the software cannot change its value. In
embedded systems with RAM and ROM, const added to a global
variable means it will be allocated in ROM permanently (permanent
persistence). The global constant Size can be
accessed anywhere in the software system, but cannot be dynamically
changed.
const uint32_t Size=100;
void MyFunction6(void){
for(uint32_t i=0; i < Size; i++){
// stuff
}
}
When the qualifier const added to a
parameter it means the software cannot change its value within the
function. The parameter Size can be accessed in the
function, but cannot be dynamically changed. In this example, the
parameter Size is still passed in Register R0, with
temporary allocation and private scope.
void MyFunction7(const uint32_t Size){
for(uint32_t i=0; i < Size; i++){
// stuff
}
}
A static function has reduced scope. On an
embedded system, all functions are permanentally allocated in ROM. If we
add static to a function definition, the scope can be reduced to
file in which it is defined. This means only functions also defined in
this file can call it. Other names for reduced scope functions are private
functions and helper functions. In general, it is good
design to reduce scope of data and functions as much as possible.
Prototypes for public functions are placed in the header file,
whereas prototypes for static functions are not placed in the
header file. This way we can separate what a module does (by calling public
functions) from how it works (implementation of all functions
including static functions). In the following example, the
function rand is static, so it is callable within the
file. On the other hand, the function Random is
public and can be called from anywhere.
uint32_t static M=1;
uint32_t static rand(void){
M = 1664525*M+1013904223;
return(M);
}
uint8_t Random(void){
return(rand()>>24);
}
: How do you create a local variable in C?
: How do you create a global variable in C?
: Considering scope and allocation, what changes and what doesn't change when you add static to an otherwise global variable?
: Considering scope and allocation, what doesn't change when you add static to an otherwise local variable?
: Considering scope and allocation, what changes and what doesn't change when you add const to an otherwise global variable?
: Considering scope and allocation, what changes and what doesn't change when you add const to a function parameter?
The following video presents the implementation of local variables on the stack using SP-relative addressing
Program 6.2.1 shows the sum.c file used in the video. Program 6.2.2 shows the main.s file.
//------------Sum------------
// Input: num is a 32-bit unsigned int
// Output: Is the sum: 1+2+...+num
// Here is the C code
uint32_t Sum (uint32_t num){
uint32_t i, result=0;
for (i=1; i <= num; i++){
result += i;
}
return(result);
}
Program 6.2.1. The sum.c file used in the above video.
.text
.align 2
.global main
main:
// Call the non-recursive implementation with locals on stack
MOVS R0, #10
BL Sum // R0 should return as 55: 1+2+3...+10
Loop: B Loop // Loop forever
//------------Sum------------
// Input: R0 has input number (num)
// Output: R0 has the output which is the sum: 1+2+...+num
// Here is the Assembly Code
.equ i,0 // *Binding*: Local variable i is at offset 0 w.r.t SP
.equ result,4 // Local variable result is at offset 0 w.r.t SP
Sum:
PUSH {R4,R5,LR} // push things we will use for
scratch
SUB SP,#8 // *Allocation*: Allocate space for
// 2 local variables
both 32-bit
MOVS R4, #0
STR R4,[SP,#result] // *Access* Initialize
Result on stack
MOVS R4, #1
STR R4,[SP,#i] // *Access*
Initialize index i on stack
LoopS:
LDR R4,[SP,#i] // *Access*
load i into R4 from Stack
CMP R4,R0
BHI DoneS
LDR R5,[SP,#result] // *Access* load result
into R5 from Stack
ADDS
R5,R4 //
Result = Result + i;
STR R5,[SP,#result] // *Access* store result
from R5 to Stack
ADDS R4,#1 // i++
STR R4,[SP,#i] //
*Access* store i from R5 to Stack
B LoopS
DoneS
LDR R0,[SP,#result] // *Access* load Result in
R0 from Stack
ADD
SP,#8 //
*DeAllocation* Deallocate space for locals
POP {R4,R5,PC} //
Restore scratched registers and set pushed
// LR to PC to return
Program 6.2.2. The main.s file used in the above video. This is Cortex M0 code.
Video 6.2.2. Debugging Locals in assembly.***needs recording***
The following assembly code shows the PUSH and POP instructions can be used to store temporary information on the stack. If a subroutine modifies a register, it is a matter of programmer style as to whether or not it should save and restore the register. According to AAPCS a subroutine can freely change R0,R1,R2,R3 and R12, but the subroutine must save and restore any other register it changes. In particular, if one subroutine calls another subroutine, then it must save and restore the LR. In the following example, assume the function modifies Register R0, R4, R7 and calls another function. The programming style dictates registers R4, R7, and LR be saved. Notice the return address is pushed on the stack as LR but popped off into PC. When multiple registers are pushed or popped, the data exist in memory with the lowest numbered register using the lowest memory address. In other words, the registers in the {} can be specified in any order, but the order in which they appear on the stack is fixed. According to AAPCS we must push and pop an even number of registers. Of course remember to balance the stack by having the same number of pops as pushes.
Func: PUSH {R4,R5,R7,LR} // save registers as needed
// 1) allocate local variables
// 2) body of the function, access local
variables
// 3) deallocate local variables
POP {R4,R5,R7,PC} // restore registers and
return
The ARM processor has a lot of registers, and we appropriately should use them for temporary information such as function parameters and local variables. However, when there are a lot of parameters or local variables, we can place them on the stack. Program 6.2.3 has a large data buffer that is private to this function. It is inconvenient to store arrays in registers. Rather it is appropriate to place the array in memory and use indexed addressing mode to access the information. Because this buffer is private and temporary we will place it on the stack. 1) The SUB instruction allocates 10 32-bit words on the stack. Figure 6.2.1 shows the stack before and after the allocation. 2) During the execution of the function, the SP points to the first location of data. The local variable i is held in R0. R1 will contain i*4 as an offset into the buffer, because each buffer entry is 4 bytes. R2 will be SP+4*i. The addressing mode [R2] accesses data on the stack without pushing or popping. 3) The ADD instruction deallocates the local variable, balancing the stack.
|
Set: SUB SP,SP,#40 // 1)allocate 10
words |
// C language implementation |
Program 6.2.3. Allocation of a local array on the stack.
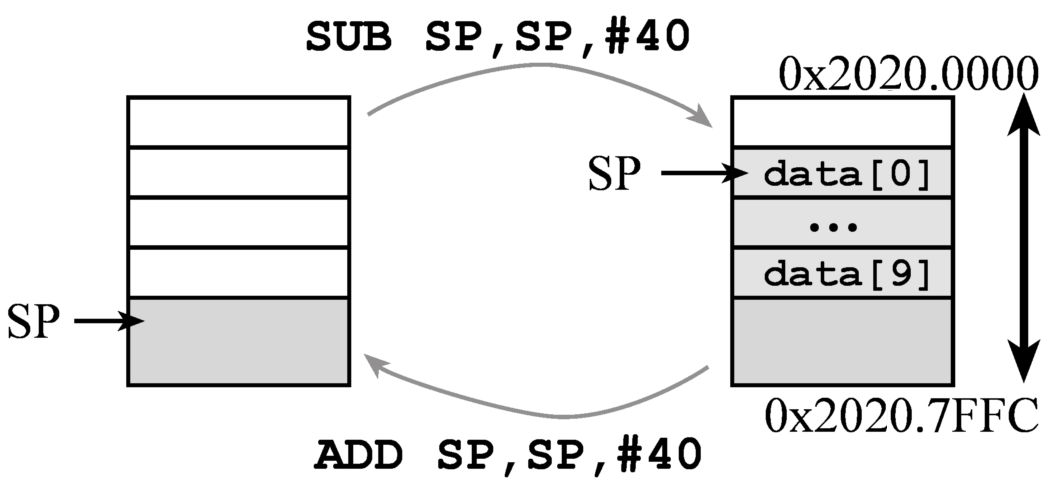
Figure 6.2.1. Allocation of a local array on the stack.
Stack implementation of local variables has four
stages: binding, allocation, access, and deallocation. In this section,
the software will create two local variables called sum and i.
1. Binding is the assignment of the address (not value) to a
symbolic name. In other words, we assign offsets for the variables. In
general, we perform binding by drawing a stack picture and deciding the
order of the local variables, see Figure 6.2.2. The symbolic name will be
used by the programmer when referring to the local variable. The assembler
binds the symbolic name to a stack index, and the computer calculates the
physical location during execution. In the following example, the local
variable sum will be at address SP+0, and the programmer
will access the variable using [SP,#sum] addressing. Similarly,
the local variable i will be at address SP+4, and the
programmer will access the variable using [SP,#i] addressing:
.equ sum,0 // 32-bit local variable, stored on the
stack
.equ i,4 // 32-bit local variable,
stored on the stack
2. Allocation is the generation of memory storage for the local variable, or assigning space. The computer allocates space during execution by decrementing the SP. In this first example, the software allocates the local variable by pushing a register on the stack. The variable sum is initialized to 0 and the variable i is initialized to 16. According to AAPCS, we must allocate space in multiples of 8 bytes. The contents of the register become the initial value of the variable.
MOVS R0,#0
MOVS R1,#16
PUSH {R0,R1} // allocate and initialize two
32-bit variables
Rather than creating local variables with initialization, the software could allocate the local variables by decrementing the stack pointer. Allocating locals this way creates them uninitialized. This method is most general, allowing the allocation of an arbitrary amount of data.
SUB SP,#8 // allocate two 32-bit variables
3. The access to a local variable is a read or write operation that occurs during execution. Because we use SP addressing with offset, we will only use LDR and STR to access local variables on the stack. In the first code fragment, we will add the contents of i to the local variable sum.
LDR R0,[SP,#i] // R0=i
LDR R1,[SP,#sum] // R1=sum
ADDS
R1,R0 // R1=i+sum
STR R1,[SP,#sum] // sum=i+sum
In the next code fragment, the local variable sum is divided by 16.
LDR R0,[SP,#sum] // R0=sum
LSRS R0,R0,#4
STR R0,[SP,#sum] // sum=sum/16
4. Deallocation is the release of memory storage for the location variable. This step frees up space. The computer deallocates space during execution by incrementing SP. The software deallocates two local variables by incrementing the stack pointer. When deallocating, we must balance the stack. I.e., we add to the SP exactly the same number as we decremented during allocation.
ADD SP,#8 // deallocate sum
Figure 6.2.2. Allocation of two local variables on the stack.
Program 6.2.4 shows a C and assembly function implementing the same function. This assembly implementation uses the PUSH instruction to allocate and initialize the local variables.
|
Calculate: |
// C language implementation |
Program 6.2.4. Allocation of two local variables on the stack.
: Write code that allocates four 32-bit local variables, uninitialized.
: Write code that binds four 32-bit local variables to the names a,b,c,d such that a is on top.
: Assuming the name of a 32-bit local variable is b, write code that sets b to 5.
: Write code that deallocates four 32-bit local variables.
: Assume Register R0 contains the size in 32-bit words of an array, determined at run-time. Write assembly code to allocate the array on the stack.
6.3. Stack frames
Each time a function is called a stack frame is created. There are four types of data that may be saved in the stack frame. By convention, if there are more than 4 input parameters, additional parameters above 4 will be pushed on the stack by the calling program. If the function calls another function, the LR (return address) must be pushed on the stack. By convention if the function uses registers R4–R11, it will push them on the stack so their values are preserved. Lastly, the function may allocate local variables on the stack.
Video 6.3.1. Local variables using a stack frame.
Each time a function is called a stack frame is created. There are four types of data that may be saved in the stack frame. By convention, if there are more than 4 input parameters, additional parameters above 4 will be pushed on the stack by the calling program. If the function calls another function, the LR (return address) must be pushed on the stack. By convention if the function uses registers R4–R11, it will push them on the stack so their values are preserved. Lastly, the function may allocate local variables on the stack.
One limitation of SP indexed addressing mode to access local variables is the difficulty of pushing additional data onto the stack during the execution of the function. In particular, if the body of the function pushes additional items on the stack, the symbolic binding becomes incorrect. There are two approaches to this problem. First, we could recompute the binding after each stack push/pop. Second, we could assign a second register to point into the stack. To employ a stack frame pointer we execute the initial steps of the function: saving LR, saving registers, and allocating local variables on the stack. Once these initial steps are complete, we set another register to point into the stack. Because R4–R7 will be saved and restored any of these would be appropriate for the stack frame pointer. E.g.,
MOV
R7,SP
We will not consider using R8-R12 as stack frame pointers on the Cortex M0, because these registers cannot be used for indexed mode addressing.
This stack frame pointer (R7) points to the local variables and parameters of the function. It is important in this implementation that once the stack frame pointer is established (e.g., using the MOV R7,SP instruction), that the stack frame register (R7) not be modified. The term frame refers to the fact that the pointer value is fixed. If R7 is a fixed pointer to the set of local variables, then a fixed binding (using the .equ pseudo op) can be established between Register R7 and the local variables and parameters, even if additional information is pushed on the stack. Because the stack frame pointer should not be modified, every subroutine will save the old stack frame pointer of the function that called the subroutine and restore it before returning. Local variable access uses indexed addressing mode using Register R7.
|
.equ sum,0 |
// C language implementation |
Program 6.3.1. Allocation of two local variables using a stack frame.
: When should we use stack frames with R7 addressing instead of regular local variables with SP addressing?
: When implementing stack frames with R7 addressing, do we subtract from R7 or from SP when allocating local variables?
6.4. Linking C to assembly
One of the advantages of ARM Architecture Procedure Call Standard (AAPCS) is that we can write one function in one environment (C or assembly) and invoke it from another environment. Recall the rules of AAPCS:
- Pass input parameters in R0, R1, R2, R3 as needed.
- Return output parameter in R0 if needed.
- The function can freely use R0,R1,R2,R3,R12.
- The function can use R4–R11, but save them at beginning and restore them at the end.
Video 6.4.1. Linking C to assembly.***needs recording***
In the following example, Program 6.4.1, the C function on the left calls an assembly function on the right. C needs a function prototype. Normally we put function prototypes in a separate header file. However in this example, the prototype is simply placed above the C program. In the assembly file, we specify the assembly function as public by exporting its address using .global pseudo-op.
|
// C program that invokes |
// low level assembly |
Program 6.4.1. C program calls an assembly function.
In this next example, Program 6.4.2, the assembly function on the left calls a C function on the right. There is no need for a prototype for an assembly language to call a C function; both do need to follow AAPCS. The C compiler automatically creates AAPCS-compliant code. To link the C function into the assembly file, we use the .global pseudo-op inside the assembly file. In the C file, we simply define the function.
|
// Assembly program that invokes |
// low level C |
Program 6.4.2. C program calls an assembly function.
Notice the C version of sqrt is quite different than the assembly version. The C code uses Newton's Method, which is based on ancient Babylonion math dating back to 1000 BCE. If you were to calculate the sqrt(2,500,000,000) = 50,000, the assembly version will iterate 50,000 times, while the C version takes just 16 interations. Newton's Method will give on one bit per loop. For more information see, Square Roots via Newton's Method, by S. G. Johnson, MIT Course 18.335.
: Why do we write assembly language functions using AAPCS?
: Think about which registers do not have to be saved/restored, and which registers must be saved/restored according to AAPCS. . Think about which registers are automatically pushed on the stack when an interrupt is processed. What does this mean?
6.5. Serial Peripheral Interface (SPI)
Serial Peripheral Interface (SPI) is a synchronous serial protocol. Serial means data is transmited on a single line, one bit at a time. Synchronous means the protocol also includes a clock, see SCK in Figure 6.5.1. In its simplest form, SPI connects one controller (also called master) to one peripheral (also called slave). PICO (peripheral in controller out) is a serial line transmitting data from controller to peripheral. Another name for PICO is master out slave in (MOSI). Data can flow in both directions at the same time (called full duplex). POCI (peripheral out controller in) is a serial line transmitting data from peripheral to controller. Another name for POCI is master in slave out (MISO). The SPI protocol also includes a chip select (CS), which is driven low by the controller during a transmission. The peripheral will interact with a transmission if its chip select is low. Chip select is negative logic, meaning the inactive state is high, and the active state is low.
Figure 6.5.1. The four signals that comprise SPI.
One edge of the clock is used by the transmitter to change the data, and the other edge of the clock is used by the receiver to read the data. This way the data is stable when the receiver reads it. In Figure 6.5.2, T marks the time the controller changes the output pin. The DA intervals shows when the data output (PICO) is available or valid. R marks the time the peripheral reads the pin. The DR intervals shows when the data required to be valid. To operate correctly, the DA interval must overlap (start before and end after) the DR interval.
Figure 6.5.2. Data output and data input are synchronized to the clock.
Observation Synchronous protocols are fast and reliable.
: In Figure 6.5.2, the rising edge of the clock stores PICO into the peripheral. What is the definition of set up time?
: What is the definition of hold time?
: Define the data required interval in terms of the clocking edge, the set up time, and the hold time.
The SPI protocol sends 8 to 16 bits in a transmission. The interface to the ST7735R display utilizes an 8-bit frame, see Figure 6.5.3. The CS goes low, 8 bits are transmitted synchronized to 8 pulses on SCK, and then CS goes high.
Figure 6.5.3. One frame transmits 8 bits of data.
: What is the order of the bits sent serially with SPI?
The SPI protocol bidirectional transmission. We classify it as full duplex because data flows in both directions at the same time. The SPI interface supported two shift registers, one in the controller and a second in the peripheral. Both shift registers are clocked at the same time, using one edge to shift the data out and the other edge to shift the data in, see Figure 6.5.4.
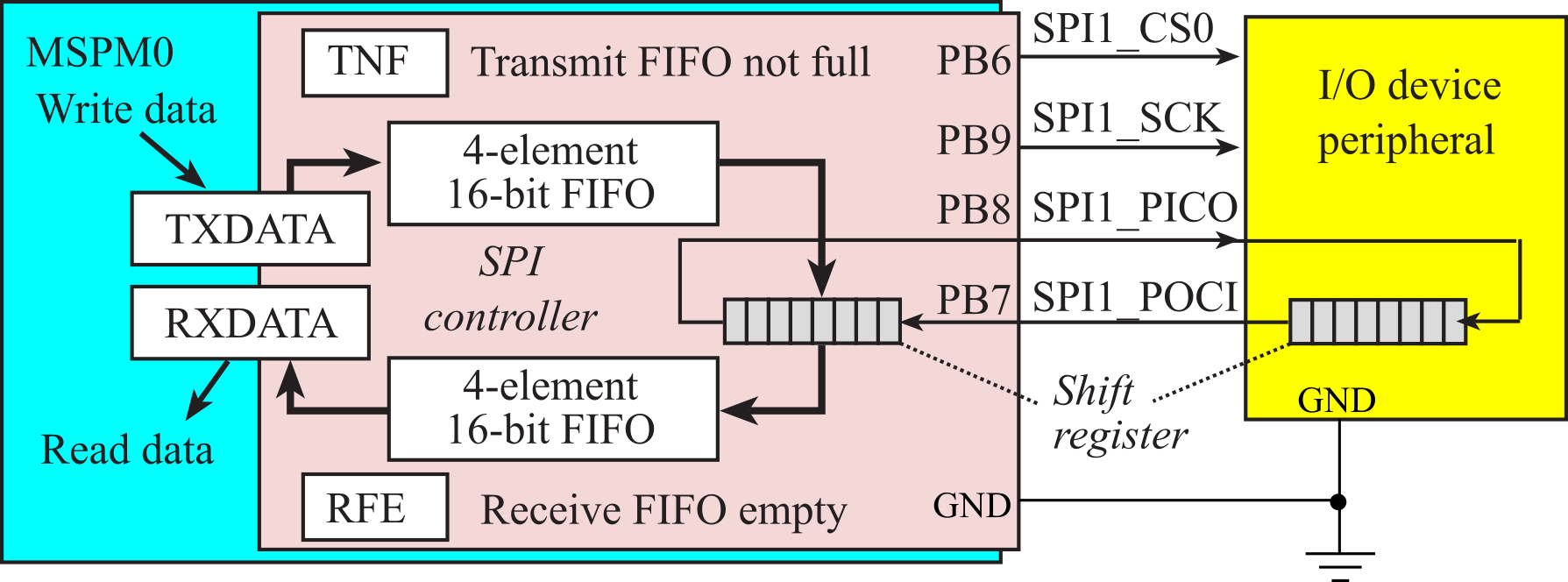
Figure 6.5.4. The SPI protocol exchanges the data in the two shift registers.
: Explain how SPI is full duplex?
In the following 8-Bit SPI Interactive, we are examining how an SPI bus would function. Additionally we want to examine how different factors such as the clock polarity(CPOL) and clock phase(CPHA) can affect how we are reading/interpretting the data produced.
: What makes this protocol both fast and reliable?
6.6. ST7735R Interfacing
In this section we will interface a ST7735R LCD using SPI protocol. The interface to the ST7735R will be classified as simplex because data will only flow from controller to peripheral. Figure 6.6.1 shows the interface to the Adafruit LCD Connections for other ST7735R LCDs can be found in the starter code for this class.
Figure 6.6.1. MSPM0G3507 interfaced to the Adafruit ST7735R LCD.
Figure 6.6.2. shows the 128 by 160 pixel color display
Figure 6.6.2. ST7735R display with 160 by 128 16-bit color pixels.
Video 6.6.1. Interfacing the ST7735R LCD.
: How does the ST7735R software driver specify color?
Before we output data or commands to the display, we will check a status flag and wait for the previous operation to complete. Busy-wait synchronization is very simple and is appropriate for I/O devices that are fast and predicable. D/C stands for data/command; you will make D/C high to send data and low to send a command. Because the LCD is so fast we will use "busy-wait" synchronization, which means before the software issues an output command to the LCD, it will wait until the display is not busy. In particular, the software will wait for the previous LCD command to complete.
: What does the D/C pin do?
: What does the TFT_CS pin do?
: What does the MOSI pin do?
: What does the SCK pin do?
Video 6.6.2. Synchronizing software to hardware.
The following pseudo-code and Figure 6.6.3 shows the steps to interact with the LCD using the SPI module. The SPI module uses a first in first out (FIFO) queue built into the hardware. Bit 4 of the SPI1->STAT register is busy. If busy is 1, it means it cannot accept another command at this point. If busy is 0, it means it ready and can accept another command. Bit 1 of the SPI1->STAT register is TNF, which stands for transmitter FIFO not full. If TNF is 0, it means the transmitter FIFO is full and it cannot accept another data output at this point. If TNF is 1, it means the FIFO is not full and can accept another data output. Notice that this interface will wait before and after each command, however multiple data outputs can occur as long as there in room in the FIFO.
writecommand: Involves 6 steps performed to send 8-bit Commands to the
LCD
1. Read SPI1->STAT and check bit 4,
2. If bit 4 is high, loop back to step 1 (wait for BUSY
bit to be low)
3. Clear D/C=PA13 to zero (D/C pin configured for COMMAND)
4. Write the command to SPI1->TXDATA
5. Read SPI1->STAT and check bit 4,
6. If bit 4 is high loop back to step 5 (wait for BUSY bit
to be low)
writedata: Involves 4 steps performed to send 8-bit Data to the LCD:
1. Read SPI1->STAT and check bit 1,
2. If bit 1 is low, loop back to step 1 (wait for TNF bit
to be one)
3. Set D/C=PA13 to one (D/C pin configured for DATA)
4. Write the 8-bit data to SPI1->TXDATA
Figure 6.6.3. Busy-wait synchronization is used to send commands and data to the display.
: What does busy-wait mean?
At the lowest level each ASCII character is mapped to an image. This mapping is called a font. The following figure and program shows how the character '6' is created on the screen as a 5 by 8 pixel image. The driver automatically inserts one blank line in between characters, so each character requires 6 by 8 pixels on the screen.
Figure 6.6.4. ST7735R character font is 5 wide by 8-tall pixels.
static const uint8_t Font[] = {
0x00, 0x00, 0x00, 0x00, 0x00, // 0x00
0x3E, 0x5B, 0x4F, 0x5B, 0x3E, // 0x01
...
0x3C, 0x4A, 0x49, 0x49, 0x31, // 0x36= '6'
...
0x00, 0x00, 0x00, 0x00, 0x00 // 0xFF
};
Program 6.6.1. ST7735R character font is 5 wide by 8-tall pixels.
There is one image for all 8 bit possibilities from 0 to 0xFF. To handle extended ASCII, which are the values 0x80 to 0xFF, make sure to change the compiler settings to select unsigned for the char type. Execute Project->Options, in the C/C++ tab deselect the box "Plain char is signed", making char unsigned.
: How many characters can fit across one row of the LCD screen?
: The ST7735R software driver uses 10 pixels in the vertical direction for each row of characters. How many rows of characters can fit on the LCD screen?
There is a rich set of graphics functions available for the ST7735R, allowing you to create amplitude versus time, or bit-mapped graphics. Refer to the ST7735R.h header file for more details.
6.7. Fixed-point Numbers
The value of a fixed point number is an integer times a constant.
The integer is stored in the computer. The constant is not stored, but it is known and fixed.
value = integer * constant
Video 6.7.1. Fixed-point numbers.
: When do we use decimal fixed point rather than binary fixed point?
: We wish to represent the sqrt(2)=1.4142135623730950488016887242097 as a decimal fixed number with a resolution of 0.001. What integer value do we use?
: We wish to represent 0.75 as a binary fixed number with a resolution of 2^-3 (1/8). What integer value do we use?
6.8. Numerical Output
Video 6.8.1. Converting integers to ASCII characters.
The Cortex M0 has a multiply instruction, MULS, but no divide. To implement numerical output of integers in decimal format, we will need division and modulus. The function in Program 6.8.1 takes two inputs and returns two outputs. It does not comply with AAPCS because it returns two values, in R0 and R1. However, we can call this function from other assembly routines.
Refer back to Section 1.7.7 for more examples of assembly functions that multiply and divide.
// Inputs: R0 is 32-bit dividend
// R1 is 16-bit divisor
// quotient*divisor + remainder = dividend
// Output: R0 is 16-bit quotient, assuming it fits
// R1 is 16-bit remainder (modulus)
udiv32_16:
PUSH {R4,LR}
LDR R4,=0x00010000 // bit mask
MOVS R3,#0 // quotient
MOVS R2,#16 // loop counter
LSLS R1,#15 // move divisor under dividend
udiv32_16_loop:
LSRS R4,R4,#1 // bit mask 15 to 0
CMP R0,R1 // need to subtract?
BLO udiv32_16_next
SUBS R0,R0,R1 // subtract divisor
ORRS R3,R3,R4 // set bit
udiv32_16_next:
LSRS R1,R1,#1
SUBS R2,R2,#1
BNE udiv32_16_loop
MOVS R1,R0 // remainder
MOVS R0,R3 // quotient
POP {R4,PC}
Program 6.7.1. 32-bit by 16-bit unsigned divide. It does not check for overflow.
For the following two checkpoints, assume R0 initially contains an unsigned integer of value n, and R1 is initially 10.
: What is the value of R0 after calling the udiv32_16 function?
: What is the value of R1 after calling the udiv32_16 function?
: Assume each instruction in udiv32_16 takes 2 bus cycles. Assume the BLO instruction never branches. Estimate execution speed of this function. Compare this speed to the 2 bus cycle time it takes to execute MULS.
: Give a mathematical equation relating the dividend, divisor, quotient, and remainder.
: Under what assumptions is this equation give a unique answer.
Video 6.8.2. Device Drivers, Successive Refinement, Number Conversions **bug at 12:38, should loop on CNT>0 and quit when CNT equals 0.**
Program 6.8.2 shows two implementations of factorial. The one on the top uses iteration, and the one on the bottom uses recursion. It is usually the case that a recursive algorithm can be rewritten in iterative form. Nevertheless, sometimes it is more convenient to implement the algorithm in recursive form.
|
// iterative implementation (22 bytes) |
// iterative implementation |
Program 6.8.1. Iterative and recursive solutions to factorial.
6.9. ECE319K Lab 6 videos
***to do***
Go to Chapter 7: Analog to Digital Conversion (ADC), Data Acquisition, and Control
This material was created to teach ECE319K at the University of Texas at Austin
Reprinted with approval from Introduction to Embedded Systems Using the MSPM0+, ISBN: 979-8852536594
Embedded
Systems - Shape the World by Jonathan Valvano and Ramesh Yerraballi is
licensed under a Creative
Commons
Attribution-NonCommercial-NoDerivatives 4.0 International License.
Based on a work at http://users.ece.utexas.edu/~valvano/mspm0/